1. The distance between two points (x₁, y₁, z₁) and (x₂, y₂, z₂) is given by the Pythagorean Theorem:

   r = √ ((x₂ - x₁)² + (y₂ - y₁)² + (z₂ - z₁)²)

2. circumference (circle) = 2 π r
   area (circle) = π r²
   surface area (sphere) = 4 π r²
   volume (sphere) = 4/3 π r³
   surface area (cylinder) = 2 π r² + 2 π r h
   volume (cylinder) = π r² h
3. For the right triangle:

   

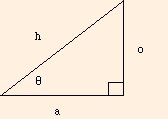
   sin θ = o / h
   cos θ = a / h
   tan θ = o / a
4. sin (a + b) = sin a cos b + cos a sin b
   cos (a + b) = cos a cos b - sin a sin b
   cos² θ + sin² θ = 1
   1 / cos² θ = 1 + tan² θ
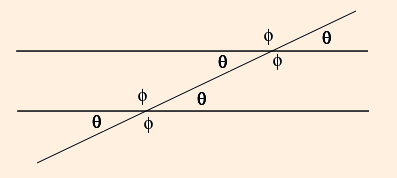
5. When two parallel lines are crossed by a transverse line, the alternate interior angles are equal:

   

(prev)

(top)

(next)

©2010, Kenneth R. Koehler. All Rights Reserved. This document may be freely reproduced provided that this copyright notice is included.