Fluids

The term fluid applies to both liquids and gases. The essential differences between fluids and solids can be summarized as follows:

  1. Fluids are shapeless. In more technical terms, we say that fluids do not resist shear. That is, it is relatively hard to bend a solid object, but a fluid splashes under the same force.

  2. When a force is applied to a fluid, the pressure increases, but whereas the force is directional, the pressure is omnidirectional. Consider the force applied to the surface of a glass of water by the atmosphere. That force is downward, but the resulting pressure is felt throughout the water. If we measure the pressure at any given depth, it is the same on all sides of the measuring instrument.

  3. Fluids are viscous. While fluids do not resist shear, they do resist changes in the rate of change of shear. The more viscous the fluid, the greater its resistance to changes in the rate of shear: colloquially, we say the fluid is syrupy. Viscosity is measured in Poise (denoted P):
    1 P = 0.1 kg / (m s).
    It is most often described in terms of cgs units: units whose fundamental measures are the gram, the centimeter and the second. In cgs units, since there are 1000 grams in a kilogram and 100 centimeters in a meter, we have
    1 P = 0.1 kg / (m s) * (1000 g / kg) * (1 m / 100 cm)
    = 1 g / (cm s).
    The viscosity of water is .01 Poise, and the viscosity of blood is .04 Poise.

  4. Fluids flowing past a solid surface obey the no slip condition: the velocity of the fluid at the solid surface is zero. This may seem surprising, until you recall that your dishwasher often does not clean well when, for instance, the dishes are coated with cheese. The no slip condition means that the water by itself is not as effective as scrubbing with a solid object, and so many of us wipe the dishes before putting them in a dishwasher. Since the velocity of the fluid is nonzero elsewhere, the no slip condition implies that a velocity gradient exists in the flowing fluid: the velocity depends on the position where it is measured. In a pipe (or blood vessel), the velocity profile across the diameter is essentially parabolic (depending on r2) and has axial (circular) symmetry:

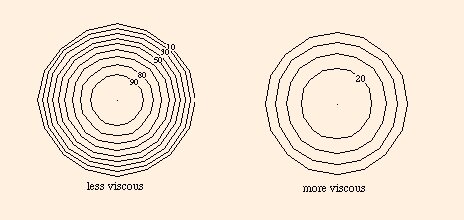
    the velocity does not depend on the angular position on any concentric circle, but only on the distance from the center.

    The fact that the velocity is constant on each circle leads us to think of the fluid as flowing in concentric sheets, but in fact the velocity is a smooth function of distance from the center. For higher viscosity, the velocity gradient is shallower. Essentially, viscosity opposes the existence of steep velocity gradients. The larger the viscosity, the gentler is the shape of the parabolic velocity gradient, and the velocity is more nearly constant across the section.

  5. Fluids are subject to turbulence. Smooth flow is called laminar, implying that layers of fluid flow past each other in a sheet-like fashion. The flow is turbulent when eddies and vortices (whirlpools) occur. Fluids lose energy through dissipation in both types of flow. During laminar flow, the viscosity causes dissipative losses between the sheets of fluid: the fluid resists changes in the velocity gradient, and that costs energy. In turbulent flow, energy is required to create the eddies and vortices, resulting in an energy loss by the fluid as a whole.

    Fluid flow in a pipe crosses the threshold from laminar to turbulent flow when a dimensionless parameter called the Reynold's Number (denoted Re) reaches about 2000. It is defined as

    Re = 2 ρ r v / η,
    where ρ denotes the density of the fluid (1.05 g / cm3 for blood), v is the fluid's velocity and η is the Greek letter eta denoting the viscosity. Re is essentially the ratio of the inertial forces (tending to keep the fluid flowing) to the viscous forces experienced by a layer of fluid. Its value indicates the relative unimportance of viscosity (ie., low Re corresponds to very viscous situations). Typical values for human arteries and veins are:
    vesselrvRe
    artery.2 cm28 cm/s294
    vein.25 cm36 cm/s472.5

Poiseuille's Equation

For laminar flow through a uniform straight pipe, the flow rate (volume per unit time) is given by Poiseuille's Equation:

F = ΔP π r 4 / 8 η l,
where ΔP is the pressure drop experienced by the fluid as a result of viscous losses along the length l of the pipe.

We would like to use Poiseuille's Equation to discuss the blood vessels in animal circulatory systems. While the flow is essentially laminar outside of the capillaries, it is pulsatile throughout the arterial subsystem: the pressure varies as a periodic function of time. In addition, the equation is based on the parabolic velocity gradient, but since pressure waves in arterial walls propagate more quickly than those in blood, the velocity profile is more uniform than parabolic. Beyond that, Poiseuille's Equation assumes a constant viscosity, whereas the viscosity of blood actually changes with velocity, since blood is not a uniform fluid. In fact, the viscosity is much lower in the capillaries than in the rest of the system, since the red blood cells line up in single file to pass through them. On top of everything else, the blood vessels are not straight, uniform pipes!

All of these reservations notwithstanding, we can apply Poiseuille's Equation to the circulatory system to understand the scaling relationships between the various parameters. For instance, an occluded artery is often the subject of angioplasty or even bypass, in order to increase the inner radius of the vessel. We can use Poisseuille's Equation to understand the functional dependency of flow on radius: since flow is proportional to the fourth power of radius, we see that increasing the radius by a factor of 2 results in an increase in the flow rate by a factor of 16. We say that the flow scales with the fourth power of the radius. In a similar fashion, we can relate any two of the variables, holding all of the others constant. Since flow is equal to velocity times cross sectional area, we can also relate the blood velocity and therefore the Reynold's number to the other parameters.

It is necessary to have a single equation which contains exactly two interrelated variables in order to deduce a scaling relationship. In the event that three variables are interrelated through two or more equations, one variable must be eliminated. Any single equation which contains three interrelated variables is insufficient to deduce the relation; in that case a different equation is necessary.

Note that in this applet, the answers must be exact! Remember that answers less than one must be entered as fractions (ie., 1/2).

You need a Java-capable browser to be able to use the applets. If they do not work with your Windows system, download the Java VM (Virtual Machine) for your version of Windows at the download section at java.sun.com.

The hydrostatic pressure in a volume of fluid is the pressure at any given depth (d) due to the weight of the fluid above that depth:

P = ρ g d
This pressure is independent of any atmospheric or surface pressure exerted at depth d = 0.

. . .

Friction often presents itself as drag when an object is moving through a fluid medium. It is a force proportional to the object's velocity, but opposite to the direction of motion:

Fd = - b V.
Here b is the drag coefficient, with dimensions of mass over time. The drag coefficient depends on the radius and shape of the object, and the viscosity of the medium. For a sphere of radius r, the drag coefficient is
b0 = 6 π η r.
For other shapes, we multiply by a shape factor b / b 0 which depends on the shape of the object; for a more cylindrical object, the shape factor might be around 1.5.

Consider an object sinking in a swimming pool:

Opposing gravity is the buoyant force of the water, which attempts to raise the object, and the drag force, which slows its sinking. The buoyant force is equal to the weight of the fluid displaced by the object:

Fb = m v ρ g.
Here v is the specific volume of the object (volume per unit mass). Thus m v gives the volume of the object, and ρ g gives the gravitational force per unit volume of the fluid.

Equating the sum of the forces due to gravity, buoyancy and drag to the mass times the acceleration, and taking both V and A to be positive for a sinking object, we find

m g - m v ρ g - b V = m A
or
(1 - v ρ) m g - b V = m A
First note that the object floats if v ρ > 1, that is, if the density of the object is less than that of the fluid. If the object does sink, V is initially small, A is positive and V therefore grows. But A clearly decreases, and at some point becomes zero. At that point, V stays constant. The exact result requires calculus, but for large times relative to m / b, the velocity approaches the terminal velocity:
Vt = (1 - v ρ) m g / b.
Note that the hydrostatic pressure does not affect this result, since it exerts a force on the object equally in both the upward and downward directions.

In electrophoresis, which is used in DNA analysis and the determination of protein molecular weights, an electric field takes the place of gravity and buoyancy. The acceleration equation becomes

q E - b V = m A
and the terminal velocity is then
Vt = q E / b
The molecules rapidly reach terminal velocity, and the measurement of the velocity (or correspondingly, the distance the molecules travel in a given time) determines the charge on the molecule. Provided that the molecules have been denatured (unfolded) and charged by the addition of an anionic detergent like sodium dodecylsulfate (SDS), the charge will be proportional to the mass of the molecule and the molecular weight can be determined.

The next section builds an electrical analogy to fluid systems.

©2009, Kenneth R. Koehler. All Rights Reserved. This document may be freely reproduced provided that this copyright notice is included.

Please send comments or suggestions to the author.